Apollonius gasket
One very special formation of integer circle relations, is what is called the apollonius gasket. This structure starts with a Descartes integer formation, In this formation four circles all tangent to each other all have integer inverse radius. Then any circle tangent to any three of these, also has integer inverse radius. This also continues, as the circle tangent to any new triple tangent to each other, also integer inverse radius. The circles get smaller and smaller; the inverse radiuses always has integer values, this value increases. An explanation of this phenomena we find in the 2. Descartes theorem. Theorem: Given three circles a, b c tangent to each other, and two circles d and e tangent to the three. Then the relation of the radiuses of the circles are given by:
If the expression of the inverse radiuses are the same as the circles we get:
Given a Descartes formation, we can then from this relation calculate the polar apollonius circle, and when the other inverse radiuses are integer, then the polar also has to be so. The 2.Descartes theorem and the eight circle theorem.The 2. Descartes theorem can be considered a specie of the Eight circle dandels. When the three generating circle becomes tangent to each other, then three pair of apollonius circles falls together with them, and we arrive at the formula above. The cosine 2. Descartes theoremThe same formula that holds for the five inverse radiuses in the 2. Descartes formation, also holds for the the cosine relation to a line. Theorem: Given three circles a, b and c that are tangent to each other, and two apollonius circles d and e tangent to these. Then a relation of the cosines of the angles between these circles and any line l is given by:
Her the cosine can be written as:
The formula then becomes
This is generalized in the eight circle theorem. |
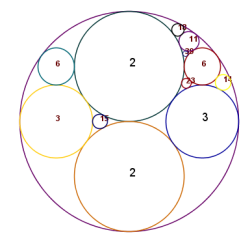
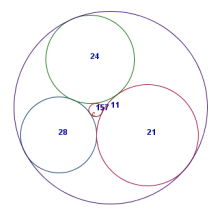
|
Demonstration
In the